
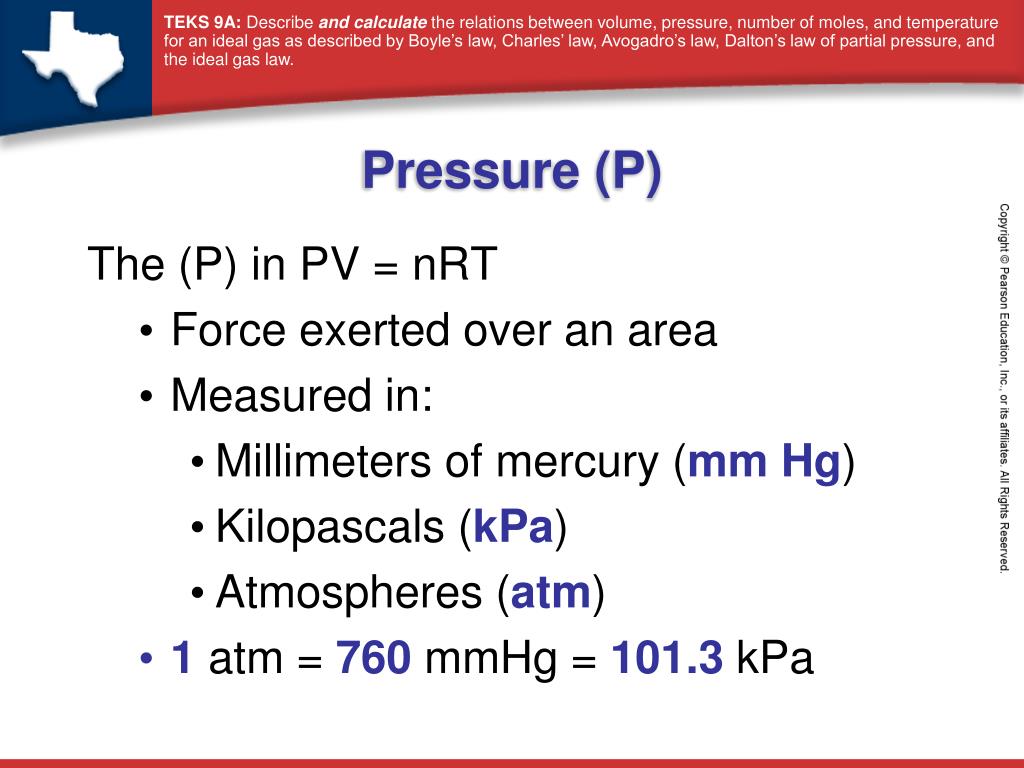
This paper is focused on the fragile nature of planetesimals. Key words: protoplanetary disks / planet–disk interactions / planets and satellites: dynamical evolution and stability Since destruction typically occurs at small stellar distances due to supersonic speeds, it is expected to boost pebble accretion in the inner protoplanetary disk as one of its main effects. After the destruction of a planetesimal, the remaining matter is continuously redistributed to the pebble reservoir of the protoplanetary disk. At all times, it is shown that only planetesimals on more or less circular orbits exist in the inner disk. We present a toy model of planetesimal motion and destruction to show the key aspects of this process. What we suggest in this work is that starting at times of viscous stirring, planetesimals on eccentric orbits could simply explode as they become supersonic in comparison to small, porous planetary bodies entering Earth’s atmosphere. However, at later times, they are also thought to provide background populations of mass that larger bodies might feed upon. They form the evolutionary steps of dust growth in their own respect. University of Duisburg-Essen, Faculty of Physics,Į-mail: pebble-sized objects and large bodies of planetesimal size both play important roles in planet formation. Tunahan Demirci, Niclas Schneider, Jens Teiser and Gerhard Wurm Astronomical objects: linking to databases.Including author names using non-Roman alphabets.Suggested resources for more tips on language editing in the sciences Punctuation and style concerns regarding equations, figures, tables, and footnotes Miller, Computational Methods of Neutron Transport, American Nuclear Society, 1993, ISBN: 2-4. Hetrick, Dynamics of Nuclear Reactors, American Nuclear Society, 1993, ISBN: 3-2. Neuhold, Introductory Nuclear Reactor Dynamics, American Nuclear Society, 1985, ISBN: 9-4. Bezella, Introductory Nuclear Reactor Statics, American Nuclear Society, Revised edition (1989), 1989, ISBN: 3-2. Department of Energy, Nuclear Physics and Reactor Theory. DOE Fundamentals Handbook, Volume 1 and 2. January 1993. Robert Reed Burn, Introduction to Nuclear Reactor Operation, 1988.Physics of Nuclear Kinetics. Addison-Wesley Pub. Nuclear and Particle Physics. Clarendon Press 1 edition, 1991, ISBN: 978-0198520467 Nuclear Reactor Engineering: Reactor Systems Engineering, Springer 4th edition, 1994, ISBN: 978-0412985317 Stacey, Nuclear Reactor Physics, John Wiley & Sons, 2001, ISBN: 0- 471-39127-1. Baratta, Introduction to Nuclear Engineering, 3d ed., Prentice-Hall, 2001, ISBN: 8-1. Lamarsh, Introduction to Nuclear Reactor Theory, 2nd ed., Addison-Wesley, Reading, MA (1983). Department of Energy, Thermodynamics, Heat Transfer and Fluid Flow. DOE Fundamentals Handbook, Volume 2 of 3. May 2016. Fundamentals of Heat and Mass Transfer. C.Fundamentals of Heat and Mass Transfer, 7th Edition.p s is stagnation or total pressure in Pa,.u is flow velocity to be measured in m/s,.Velocity can be determined using the following formula: A Pitot tube is a pressure measurement instrument used to measure fluid flow velocity. Stagnation pressure is sometimes called pitot pressure because it is measured using a pitot tube. Total and dynamic pressure are not pressures in the usual sense – they cannot be measured using an aneroid, Bourdon tube, or mercury column. Static pressure + dynamic pressure = total pressure (stagnation pressure) The simplified form of Bernoulli’s equation can be summarized in the following memorable word equation: In the high-velocity flow through the constriction, kinetic energy (dynamic pressure – ½.ρ.v 2) must increase at the expense of pressure energy (static pressure – p). This lowering of pressure in a constriction of a flow path may seem counterintuitive but seems less so when you consider the pressure to be energy density. Static pressure and dynamic pressure are terms of Bernoulli’s equation:īernoulli’s effect causes the lowering of fluid pressure (static pressure – p) in regions where the flow velocity increases. Stagnation pressure equals the sum of the free-stream dynamic pressure and free-stream static pressure.
This effect is widely used in aerodynamics (velocity measurement or ram-air intake). At a stagnation point, the fluid velocity is zero, and all kinetic energy has been converted into pressure energy (isentropically). In fluid dynamics and aerodynamics, stagnation pressure (or pitot pressure or total pressure) is the static pressure at a stagnation point in a fluid flow. In general, pressure is a measure of the force exerted per unit area on the boundaries of a substance.


 0 kommentar(er)
0 kommentar(er)
